Marangoni对胶体薄膜蒸发光刻图案的影响

I. INTRODUCTION

The solution coating of colloidal particles is frequently used in the production of displays and other optical films. A crucial step in these manufacturing processes is the drying of the coated film by evaporation to yield a particulate film of uniform thickness. The main motivation behind the present work  and  the  question  attempted  to  be  touched  upon  is whether Marangoni instabilities due to evaporation can have an effect on coated film quality and integrity. Nonuniformi- ties in colloidal particle deposits have been extensively ana- lyzed in the context of evaporating droplets because of en- hanced  evaporation  at  the  contact  lines.1–4   However,  in coated products that are essentially two-dimensional (2-D) in lateral extent, other factors rather than edge effects may be important.

Routh and Russel5 and Tirumkudulu and Russel6 study theoretically and report experimental observations on drying colloidal dispersions in the form of thin fifilms of fifinite lateral extent. In a thorough and insightful analysis they take into account several effects, such as the formation of a closely packed particle front at the periphery of the fifilm, the motion of that front towards the fifilm center, the effects of capillary pressure, which may result in a second front of dried particles following the former, as well as in deforming the particles and creating dry fifilms of very small porosity. In their analysis, the Brownian diffusion of the particles is assumed large enough so that it effectively homogenizes the particle concentration across the liquid fifilm. In a simpler setting, Routh and Zimmerman7 consider a fifilm of infifinite lateral extent, evaporating at a constant rate and analyze the effect of a nonzero particle Peclet number by solving a onedimensional ! 1-D" diffusion equation. Thus, the assumption of uniform concentration is relaxed and even at relatively low Peclet numbers a concentration gradient is predicted to appear near the interface and advance towards the substrate. In their analysis, fifilm deformation and convective motion are assumed to be absent. The present work focuses precisely on those two aspects, which may be driven by thermocapillary phenomena or other effects giving rise to surface tension gradients. Thus, the simple setting of an unbounded thin fifilm of a colloidal dispersion, as in Routh and Zimmermann is retained, but the temperature fifield and the convective motion in the fifilm due to Marangoni effects are analyzed.

A thorough review on thermocapillary phenomena is not attempted here, but only some points relevant to the subsequent discussion are highlighted. The interested reader may consult Davis,8 Oron et al., 9 Van Hook et al., 10 and references therein. Apart from the instability giving rise to cellular convection patterns fifirst analyzed by Pearson,11 a different mode of instability was predicted by Scriven and Sterling.12 Whereas in the former inertial effects play a decisive role and the fifilm thickness remains approximately uniform, the second mode appears at effectively zero Marangoni number when gravity is absent. This instability is manifested as a long-wavelength surface deformation mode that can ultimately lead to the formation of dry patches or elevated spots. When gravity is present, a fifinite Marangoni number is required, which essentially translates into relatively high heat transfer coeffificients in the gaseous phase to provide suffifi- cient cooling of the liquid interface. Thus, sophisticated experimental systems and meticulous procedures are required to obtain appropriate conditions for the instability to be observed. Van Hook et al.10,13 achieved this only relatively recently by experimenting with submillimeter fifilms of silicone oils in contact with equally thin fifilms of air or helium cooled from above. In this way a suffificient temperature gradient in the fifilm could be achieved.

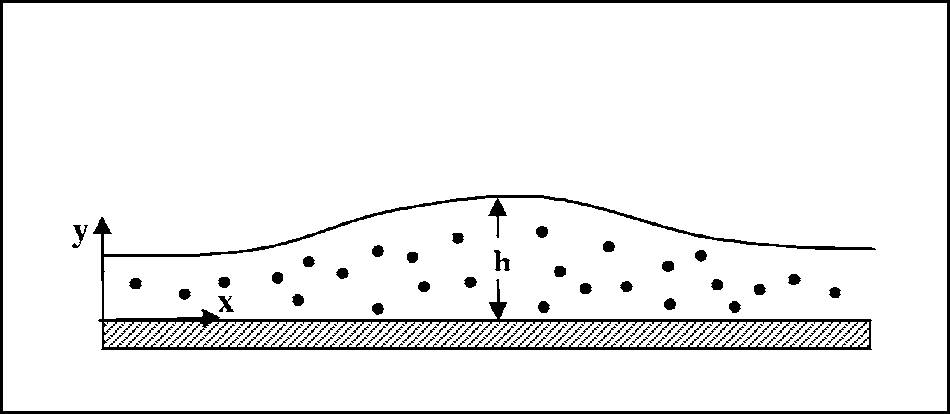
                                                        FIG. 1. Schematic of an evaporating thin fifilm of a colloidal dispersion.

II. MATHEMATICAL

 FORMULATION A. Governing equations 

A thin fifilm of a colloidal dispersion is considered on a solid substrate and in contact with ambient air, as shown in Fig. 1. The initial fifilm thickness is h0, and the particle volume fraction is ! 0. The gas phase is unsaturated with vapor and thus liquid evaporation takes place. A simple account is taken here of this phenomenon by assuming that the evaporation rate is constant and controlled by a mass transfer process in the gas phase. The evaporation rate having units of velocity may be given by E = km! pi − p" " , ! 1" where km is an appropriate mass transfer coeffificient and pi , p" are the vapor pressures at the interface and in the bulk air, respectively. Thus, in the context of previously mentioned theoretical approaches on Marangoni instability, the present one corresponds to one-layer modeling. Deviations of interfacial temperatures from equilibrium and vapor thrust effects are assumed to be negligible ! see, for example, the experimental conditions of Mancini and Maza14" . Initially, the system ! substrate, fifilm, ambient air" is considered isothermal at T0, and the substrate is assumed to be always kept at that temperature. The temperature in the fifilm changes due to evaporation. Assuming fast heat conduction and a quasi-steady state, the temperature at the interface Ti may be obtained from a balance of heat flflux through the fifilm and enthalpy of vaporization:− k! Ti − T0" h0 = # $ E. ! 2" Here, k is the fifilm thermal conductivity, $ is the density, and # is the heat of vaporization. Heat transfer from the gas face is ignored, although it could be incorporated in a manner similar to Eq. ! 1" . This issue is further discussed later in this section. It must be noted here that the thermal conductivity of the dispersion is taken to be constant and independent of particle concentration in the subsequent analysis. This necessitates imposing the constraint that the ratio of liquid to particle conductivities is a quantity of order unity.− k! Ti − T0" h0 = # $ E. ! 2" Here, k is the fifilm thermal conductivity, $ is the density, and # is the heat of vaporization. Heat transfer from the gas face is ignored, although it could be incorporated in a manner similar to Eq. ! 1" . This issue is further discussed later in this section. It must be noted here that the thermal conductivity of the dispersion is taken to be constant and independent of particle concentration in the subsequent analysis. This necessitates imposing the constraint that the ratio of liquid to particle conductivities is a quantity of order unity.