表面粗糙度对晶圆直接键合的影响

I. INTRODUCTION 

The mechanism of direct wafer bonding at room temperature has been attributed to the short range intermolecular and interatomic attraction forces, such as Van der Waals forces.1 Consequently, the wafer surface smoothness becomes one of the most critical parameters in this process. High surface roughness will result in small real area of contact, and therefore yield voids in the bonding interface. When the surface roughness exceeds a critical value, the wafers will not bond at all. The fifirst theory on the problem of closing gaps between contacted wafers was proposed by Stengl et al.2 This gapclosing theory was then further developed by Tong and Go¨sele. 1,3,4 The elastomechanics theory was used to study the energy balance between the released energy during bonding and the energy increase due to the elastic distortion of the wafer. A detailed analysis of the three-dimensional elastic fifield in the misfifit between contacted wafers has been presented by Yu and Hu very recently,5 resulting in the same results as the gap-closing theory. The gap-closing theory gives a criterion for room temperature wafer bonding. However, the extent of bonding has not been explained; and a few questions remain unclear. For example, what is the real area of contact in the wafer bonding interface after contact at room temperature? The wafer surface always possesses a random distribution of surface topography. If this is taken into account, what will be different in the theory of the surface bondability? Therefore we are motivated to develop a continuous model of the inflfluence of surface roughness on wafer bonding, that will be based on the contact mechanics theory and a statistical surface roughness model.

II. DIMENSIONAL ANALYSIS As depicted in Fig. 1

, there are basically three factors that are relevant to the wafer bonding process at room temperature and at standard atmospheric pressure: the materialdeformability, E @ N m2 2 # ; the specifific surface energy of adhesion, w @ N m2 1 # ; and the surface roughness of both wafers. The wafer surface roughness can be characterized by two variables: length parameter l @ m# , and height parameter h @ m# . Example of the length parameter is the dominant wavelength of the wafer surface topography, and that of the height parameter is the standard height deviation of the surface scan. It is clear that bonding will be easier if the surface tension is large and the material is readily deformable. So the criterion must have something to do with the ratio w/E, which has the dimension of a length. On the other hand, if the surfaces are smooth ~ h small! and the roughness has a large wavelength it is also easy to deform them. The simplest combination of h and l that gives a sensible dimensionless result is l /h2 . Therefore, we have criterion for bondability } w E l h2 . ~ 1! If the mean curvature of the asperities, b , is used to characterize the rough surface, substitution of the relation b }l 2/h ~ Ref. 6! into Eq. ~ 1! leads to criterion for bondability } w EA b h3. ~ 2! Later we will show that this is exactly the parameter for wafer bondability as derived from the contact mechanics theory

III. THEORY

All wafer surfaces are rough, at least on a microscopic scale. The scan profifile of a wafer surface is characterized by many asperities. In many situations, the asperities have spherical caps. During wafer bonding, these asperities on one wafer will fifirst make contact with the surface of the other wafer. Therefore, we shall fifirst study the contact and adhesion of single elastic spheres.

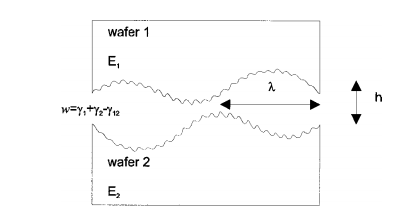
                                                                                                    FIG. 1. Cross section of two wafers in contact for direct bonding

A. The Deryagin, Muller, and Toporov theory on the contact and adhesion of single elastic spheres Let us consider the contact between an elastic sphere with high elasticity, such as silicon and a rigid flflat plane under a normal load P. The elastic sphere has a radius of R. The surface adherence forces act around the periphery of the contact area, and has a value of 2p wR, as calculated by Deryagin, Muller, and Toporon7 and Maugis.8 The distribution of the stresses within the contact regime is assumed to be Hertzian. Thus the radius of contact, a, and the elastic displacement d at the tip of the sphere are given by7,8