Chemical mechanical planarization (CMP) has been widely recognized as the most promising technology to eliminate topographic variation and achieve wafer-level (global) planarization for ultralarge-scale integrated (ULSI) circuits.2-3 Despite its extensive utilization, however, the process control of CMP remains at an empirical stage and most users still refer to the Preston equation4 as the wafer-scale material removal model. This equation states that the removal rate, RR, is proportional to the product of the polish pressure, P, and velocity, V, i.e.
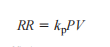
where kp is the Preston coefficient. Originally proposed for glass polishing, the Preston equation is also of an empirical nature and lacks scientific basis. Uncertainty remains regarding the basic polish behavior. Recent theoretical work and experimental evidence suggest that the Preston equation may overestimate the CMP removal rate.1,5 Tseng and Wang1 proposed an analytical model that predicts a P5/6V1/2, instead of Preston’s PV dependence of CMP removal rate
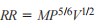
where M is the weighting factor to removal rate from other processes (e.g., slurry attack). Ouma et al.6 observed that, depending on the magnitude of the product of pressure and velocity, there exist two regimes of polish operation so that the addition of a constant term to the Preston equation yields a better fit to experimental results. Similar results have been reported elsewhere.7 Overall, the feasibility of Preston equation in describing the CMP removal rate has been challenged and deserves careful and systematic investigation. Another dilemma associated with the Preston equation is that the definition of V appears vague, since, in an orbital CMP system, the carrier and pad rotate at their respective speeds and the velocity varies from wafer center to edge during polishing. Such a speed variation has been widely observed by most CMP users, and at least two previous studies6,8 have analyzed and correlated it with other machine parameters and the polish rate. The clarification of the dependence of pressure and velocity on removal rate would be essential to the CMP process control, since, for example, simulation and prediction of topographic evolution or planarization efficiency depends strongly upon an accurate removal rate model incorporating P and V.
In this work, efforts will be attempted first to reexamine the definition and variation of velocity across a 150 mm wafer during CMP process. Simulation works will follow to investigate the impacts ofvelocity variation on polish rate, based on the original Preston equation, Tseng’s model,1 and a modified Preston equation which takes into account the deterioration in the abrasion efficiency of slurry. Comparisons (curve fitting) will be made between experimental polish data and the simulated results based on the three models. The pressure dependence of the removal rate models and other parameters (e.g., weighting factor) are evaluated. The feasibility of the three models is discussed.
Velocity Simulation
In this study, velocity is defined as the relative velocity (v) between an abrasive particle and a specific point on the wafer, during CMP operation. Several assumptions regarding the movement of wafers and abrasive particles need to be established. First, particles are all embedded in the surface features of pad. In this case, the asperity contact model9 is adopted. In this model, particles retained on the asperity of pad carry out the abrasion action. This suggests that the maximum abrasion capability of particles occurs when particles are embedded on the asperity of pad. Under this circumstance, the velocity of particles is the same as that of the pad. Second, we assume that no slipping and sliding movement occurs between the wafer and carrier during polishing so that the wafer speed is the same as the carrier speed. In addition, the wafer center coincides with the carrier center. All geometric and machine parameters are constructed based on an IPEC 372M CMP system for 150 mm wafers. Given known pad revolutions per minute (rpm) (v p), wafer rpm (v a), and the distance between pad center and wafer center (d), the relative velocity (v) on any specified point on the wafer can be deduced, using the procedure described in the Appendix.
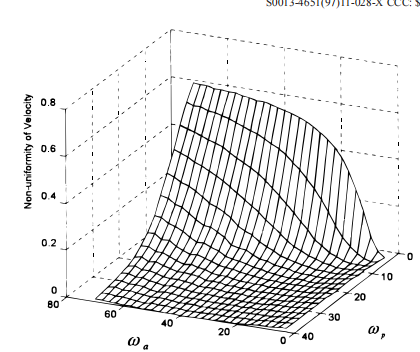
Figure 1. The correlation between NUV, v a, and vp. The distance between wafer and pad centers, d, is set at 150 mm.
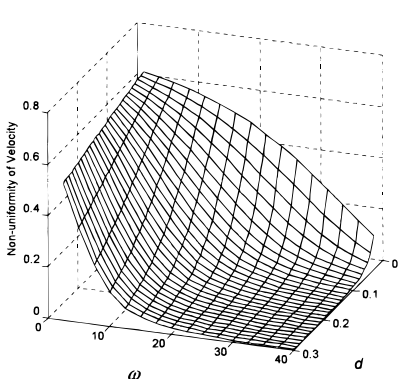
Figure 2. NUV vs. vp and d. va 5 60 rpm.
Note that the NUV considered herein may not necessarily correspond to the nonuniformity of removal rate, since the latter is a much more complex function of many other factors. Wafer warpage, back pressure, the type of carrier design, pad surface feature, and slurry hydrodynamics, to name a few, all affect removal rate uniformity to certain extent.
上一篇: 碳化硅的化学机械抛光
下一篇: 晶圆级石墨烯集成电路